Whole numbers are the cornerstones of basic mathematics, forming the foundation for all sorts of calculations, from simple counting to complex equations.
Have you ever wondered how many slices are left in that delicious pizza? Or maybe you’re curious about your high score on the latest video game? Well, the answer to both these questions, and countless others in our daily lives, lies in the fascinating world of whole numbers. This article will be your one-stop guide to understanding them, exploring their properties, and appreciating their applications in the real world. So, buckle up and get ready to dive into the wonderful world of whole numbers.
What is a Whole Number?
But what exactly are whole numbers? In simple terms, whole numbers are non-negative integers, including zero (0, 1, 2, 3, and so on). They represent whole quantities, meaning they can’t be parts of a whole or have decimal places. This distinguishes them from fractions, which represent portions of a whole (like 1/2), and decimals, which are numbers with a decimal point (like 3.14).
Here’s a helpful analogy: Imagine a basket full of apples. The number of apples in that basket is a whole number. You can’t have half an apple, or 1.5 apples, in reality. So, 0, 1, 2, 3, and so on, represent the number of whole apples in the basket.
It’s important to differentiate between whole numbers and their close cousins, natural numbers. Natural numbers are all positive integers starting from 1 (1, 2, 3, 4, and so on). The key difference is that it includes zero, while natural numbers don’t. Think of natural numbers as representing the number of items you add to an empty basket (starting from 1), while whole numbers can represent the total number of items in the basket, including the possibility of having none (zero).
Properties of Whole Numbers
Whole numbers, like any good team, have their own set of rules, or properties, that govern how they interact with each other. Let’s explore some of its essential properties:
1. Closure Property: This fancy term simply means that when you add or multiply two whole numbers, the result will always be another whole number. For example, 3 + 4 equals 7, and 5 x 2 equals 10. Both the sum and the product are whole numbers. This property ensures that you can stay within the realm of whole numbers when performing basic operations.
2. Commutative Property: This property highlights the commutative nature of addition and multiplication with whole numbers. In simpler terms, the order in which you add or multiply whole numbers doesn’t affect the final result. So, 2 + 5 is the same as 5 + 2, and 4 x 3 is the same as 3 x 4. Both operations will give you the same answer (7 and 12, respectively).
3. Associative Property: Similar to the commutative property, the associative property deals with grouping in addition and multiplication. It states that the way you group whole numbers doesn’t affect the outcome. Let’s break it down with an example: (2 + 3) + 5 is the same as 2 + (3 + 5). In both cases, the answer is 10. The same principle applies to multiplication as well: (3 x 4) x 2 is the same as 3 x (4 x 2).
4. Identity Elements: Whole numbers also have special members called identity elements. These are numbers that, when added or multiplied with any other whole number, leave the result unchanged. The additive identity for whole numbers is zero (0). No matter what whole number you add to zero, the sum remains the same (e.g., 5 + 0 = 5). Similarly, the multiplicative identity is one (1). Multiplying any whole number by one doesn’t change its value (e.g., 7 x 1 = 7).
Similarities and Differences with Natural Numbers
We’ve established that whole numbers and natural numbers are close companions, but there’s a subtle difference between them. Let’s delve deeper into their similarities and the key distinction that sets them apart.
Natural Numbers: The Counting Crew
Natural numbers are the positive whole numbers, starting from 1 (1, 2, 3, 4, and so on). They represent the concept of counting things, like the number of steps you climb or the number of apples you pick from a tree.
Similarities Between Whole and Natural Numbers:
- Both sets consist of integers, which are essentially numbers without negative signs.
- Both play a crucial role in basic arithmetic operations like addition, subtraction, multiplication, and division (although division with zero requires special considerations).
- They form the foundation for understanding more complex mathematical concepts.
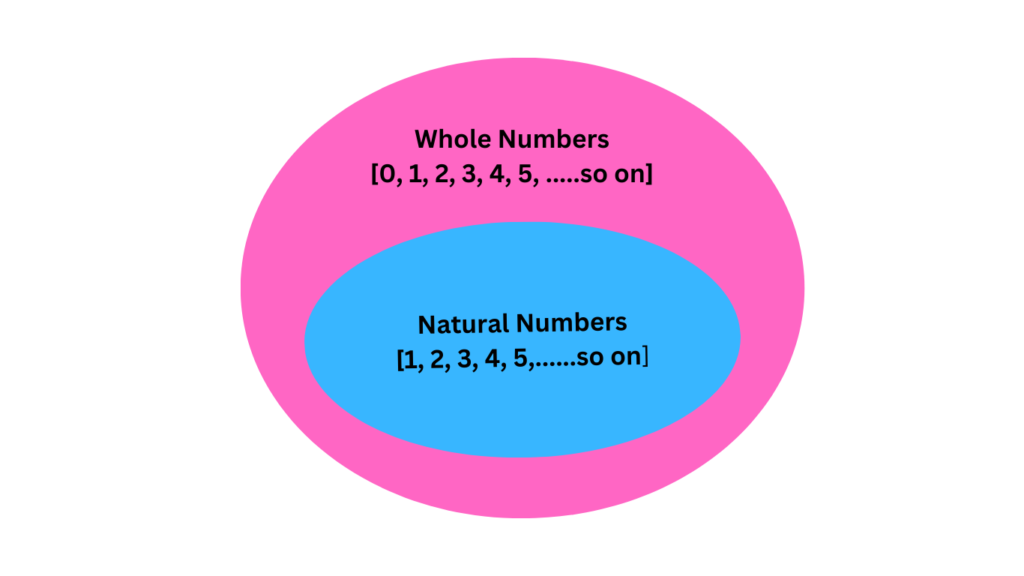
The Zero Factor: The Key Distinction
The main difference between whole and natural numbers lies in the inclusion of zero. Whole numbers encompass zero (0), while natural numbers do not. This seemingly small difference has a significant impact:
- Zero represents the absence of quantity. It allows us to express situations where there are “no apples” in the basket or “no points” scored in a game.
- Including zero expands the scope of these numbers, making them more versatile for various counting and measurement tasks.
In essence, whole numbers are like a superset that includes natural numbers (all the positive integers) along with zero.
Applications of Whole Numbers
Whole numbers aren’t just mathematical concepts; they’re the essential tools we use in countless everyday situations. Here are some real-world examples of how they come into play:
- Counting: From tracking the number of guests at a party to keeping score in a game, they are the foundation of counting objects and keeping track of quantities.
- Measurement: We use them to measure distances (e.g., walking 5 kilometers), temperatures (e.g., setting the thermostat to 20 degrees Celsius), and quantities of ingredients in a recipe (e.g., using 3 cups of flour).
- Financial Transactions: From calculating your grocery bill (adding item prices) to tracking your savings (monitoring your bank balance), they are essential for managing finances.
- Inventory Management: Businesses rely on these numbers to track their stock (number of items in stock) and plan restocking needs.
- Timekeeping: We use these numbers to express hours, minutes, and seconds, helping us schedule appointments and manage our time efficiently.
These are just a few examples, but they permeate every aspect of our lives, making them truly fundamental mathematical building blocks.
Fun Facts and Curiosities
Whole numbers might seem straightforward, but there’s a surprising amount of intrigue and hidden patterns within them. Let’s explore some fascinating facts and curiosities about them:
- Prime Numbers: These are special whole numbers greater than 1 that are only divisible by 1 and themselves. Examples include 2, 3, 5, 7, 11, and so on. Prime numbers play a crucial role in cryptography, computer science, and number theory.
- Perfect Numbers: These are whole numbers that are equal to the sum of their proper divisors (all the positive divisors except the number itself). For example, 6 is a perfect number because 1 + 2 + 3 = 6. Finding perfect numbers is an ongoing mathematical pursuit, with only a few known examples existing.
- Fibonacci Sequence: This famous sequence starts with 0 and 1, where each subsequent number is the sum of the two preceding numbers (0, 1, 1, 2, 3, 5, 8, 13, and so on). The Fibonacci sequence has remarkable applications in nature, art, and even computer science.
Divisibility Rules: Here’s a handy trick for whole numbers. Divisibility rules help you quickly determine if these numbers are divisible by another number (like 2, 3, 5, or 9) without performing long division. These rules are based on patterns in the digits of the number.
For example, a whole number is divisible by 2 if the last digit is even (0, 2, 4, 6, or 8). Similarly, a whole number is divisible by 3 if the sum of its digits is divisible by 3 (e.g., 21 is divisible by 3 because 2 + 1 = 3, which is divisible by 3). These are just a few examples, and exploring divisibility rules can be a fun way to discover hidden patterns within whole numbers.
Importance in Mathematics
Whole numbers are more than just counting tools; they serve as the foundation for building a strong mathematical understanding. Here’s why they are so crucial:
- Building Blocks for Arithmetic: They are the essential ingredients for performing basic arithmetic operations like addition, subtraction, multiplication, and division. Mastering these operations with whole numbers paves the way for tackling more complex calculations.
- Gateway to Algebra: The concepts of variables and expressions in algebra heavily rely on the foundation of these numbers. Understanding how to manipulate them allows you to solve algebraic equations and work with more abstract mathematical concepts.
- Number Theory: This branch of mathematics delves into the properties and relationships between numbers. These numbers are the starting point for exploring divisibility, primality, and other fascinating number theoretic concepts.
By mastering them, you unlock the door to a vast and exciting world of mathematical exploration. They are the building blocks for higher-level math, allowing you to solve problems, analyze data, and unlock the power of mathematical thinking.
Conclusion
Throughout this article, we’ve explored the world of whole numbers, uncovering their definition, properties, and applications. We’ve seen how these fundamental numbers are not just for counting objects but are essential tools for navigating everyday life and delving deeper into the world of mathematics.
Remember, they are the foundation upon which all other numbers are built. From the simple act of counting to the intricate calculations of advanced mathematics, they play a vital role. So, the next time you count something, measure a distance, or manage your finances, take a moment to appreciate the enduring magic of whole numbers.
Frequently Asked Questions (FAQs)
Can a negative number be a whole number?
No, whole numbers are non-negative integers. They include zero and all positive integers (1, 2, 3, and so on). Negative numbers are not part of the set of whole numbers.
What is the difference between whole numbers and integers?
Integers include all positive whole numbers, zero, and negative whole numbers. In simpler terms, whole numbers are a subset of integers (all whole numbers are integers, but not all integers are whole numbers).
How are whole numbers used in geometry?
While geometry deals with shapes and their properties, whole numbers play a role in expressing measurements like lengths, widths, and areas. For example, you might use whole numbers to represent the side lengths of a square or the number of sides in a polygon.